Yes, the Common Core DOES tell teachers HOW to teach
After reading the interview of Mitch Daniels in the New York Times, I was surprised that he claims the standards are only set goals for the kids to master and that it doesn’t prescribe the methods or curriculum, that is up to the local schools and teachers. He gave an analogy using the high jump:
“I always tell people if you go to a track meet and the high jump pit, one coach can teach the flop, and one can teach the barrel roll. And if somebody wants to do the old-fashioned scissors, that’s fine too. But we’re going to have one bar. You don’t let everybody set the bar where they want.”
I like this analogy, but it doesn’t describe what the Common Core accomplishes. Here’s how his analogy really looks under Common Core; Yes, there is only one bar set, but it’s lower than what many states had and way lower than our international competition. A coach could teach the flop, or barrel roll or scissor approach if they like. However, the jumper would be disqualified in competition if he used the scissor approach because it isn’t aligned with the new standards. The Common Core sets a uniform low bar while putting new technical requirements on the approach. The highest jumper might not win, because he used a traditional approach that is no longer allowed.
Within the standards there are several pedagogical approaches that are dictating how students are to learn mathematics. In fact, the writers of the CC math standards wrote documents for teachers, publishers and curriculum developers to use to implement the standards with the “letter and spirit” intended. This is why every Common Core aligned textbook is using the same strategies and curriculum to teach the standards and it absolutely tells teachers HOW to teach.
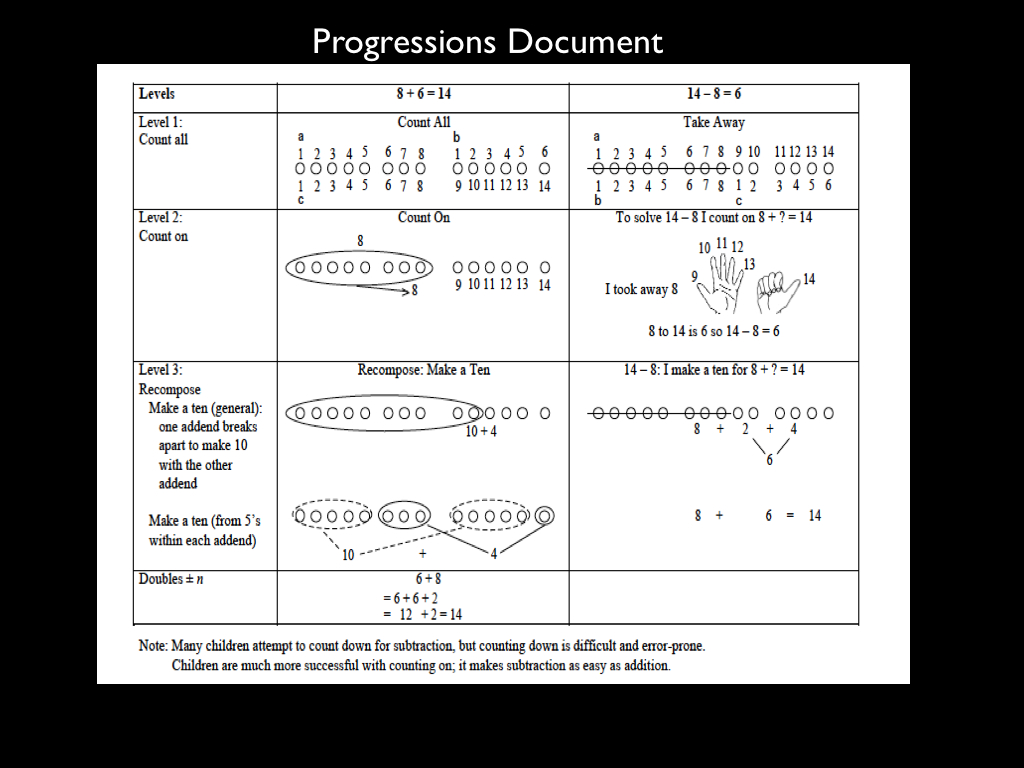
In the Progressions for the Common Core in Mathematics, the writers of the standards tell teachers EXACTLY how to teach math operations like addition, subtraction, multiplication and division. They give explicit directions how to teach it and be aligned with the spirit of the Common Core. Keep in mind that these same directions were given to the testing consortium that are formulating the items by which all teachers and students will be measured.
Here is an example of a standard for addition:
CCSS.Math.Content.1.OA.C.6 Add and subtract within 20, demonstrating fluency for addition and subtraction within 10. Use strategies such as counting on; making ten (e.g., 8 + 6 = 8 + 2 + 4 = 10 + 4 = 14); decomposing a number leading to a ten (e.g., 13 – 4 = 13 – 3 – 1 = 10 – 1 = 9); using the relationship between addition and subtraction (e.g., knowing that 8 + 4 = 12, one knows 12 – 8 = 4); and creating equivalent but easier or known sums (e.g., adding 6 + 7 by creating the known equivalent 6 + 6 + 1 = 12 + 1 = 13).
Just by reading the standard it seems obvious certain methods are being required. Teachers do not have the freedom to use other methods and getting the correct answer will not be enough. Students must be able to use these methods in order to fulfill the standard. Taking a quick look at reform math textbooks like Everyday Math one can see these are strategies they use. Note that the traditional method of the standard algorithm is not mentioned here and is not an acceptable approach to meet the standard.
Not only is the standard itself a clear indicator of how to teach addition and subtraction, but writers of the math standards elaborate on the specifics in the Progressions of the Common Core Math Standards with pictures and details:
The standards and implementation documents continue to dictate methods through out the grade levels. Here are the some of the standards for performing operations in second, third and fourth grade:
CCSS.Math.Content.2.NBT.B.7 Add and subtract within 1000, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method. Understand that in adding or subtracting three-digit numbers, one adds or subtracts hundreds and hundreds, tens and tens, ones and ones; and sometimes it is necessary to compose or decompose tens or hundreds.
CCSS.Math.Content.3.NBT.A.2 Fluently add and subtract within 1000 using strategies and algorithms based on place value, properties of operations, and/or the relationship between addition and subtraction.
CCSS.Math.Content.3.NBT.A.3 Multiply one-digit whole numbers by multiples of 10 in the range 10–90 (e.g., 9 × 80, 5 × 60) using strategies based on place value and properties of operations.
CCSS.Math.Content.4.NBT.B.5 Multiply a whole number of up to four digits by a one-digit whole number, and multiply two two-digit numbers, using strategies based on place value and the properties of operations. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
CCSS.Math.Content.4.NBT.B.6 Find whole-number quotients and remainders with up to four-digit dividends and one-digit divisors, using strategies based on place value, the properties of operations, and/or the relationship between multiplication and division. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
I have put in bold the methods they are emphasizing. These strategies are the center point of the standards. In the Publishers’ Criteria, written by the authors of the Common Core, they write that these are the focus standards and should comprise 75% of class instruction time. They do not require a traditional algorithm to be used until later grades. Answering with the traditional algorithm will not be allowed as meeting these standards.
Here is an example of how teachers are suppose to teach 6X4 using these strategies and be in alignment with Common Core. This is also how they will be assessed.
Level 1: draw six sets of four objects and count them out.
Level 2: Skip count by six four times. Student will say the multiples of six while nodding head four times or using fingers to keep track of how many multiples.
Level 3:
- They recompose the factors to be easier to use. 4×6=4x(2×3)=(4×2)3=8X3. That’s three eight times, count by threes with the head nods. This is an example of using properties of operations to solve and the count by method.
- If the problem was 132+232. They would recompose the number to make 100+30+2 and 200+30+2. Add the hundreds 300, add the tens 60, add the ones 4, then add together. This is an example of using place value to solve.
I’m sorry, but that is looking pretty FUZZY. The child doesn’t know 6X4=24, but they require him to use the abstract properties of algebra like the Distributive Property, Associative Property and Substitution Property to solve the problem? This is for third grade. They are 8 or 9 years old. This abstract processing is unnecessary for kids this age.
Most people would say teach them the fundamentals and stop the romantic notions of eight year olds thinking like mathematicians. It just isn’t appropriate and it prevents them from gaining the early knowledge and practice they need with math facts.










I’m sorry, Mr. Daniels, you are highly mistaken. Please know what you are talking about before your ignorance harms our children more than they already are.
Would you argue the same about CCSS ELA? It’s interesting to me the criticism waged against CCSS ELA when the IN Standards include many of the same expectations, particularly with respect to literary criticism, informational text and opinion writing. The difference is that PARCC/SB are poised to assess skills like opinion writing whereas ISTEP+ only assesses narrative writing. Where is the outrage over this? Internationally-benchmarked standards are only as good as are the assessments that are used to gauge progress. What happens to a great standard when teachers know it doesn’t show up on ISTEP+? If we are so against the use of technical manuals, shouldn’t we be directing our outrage toward the current IN document where the standards reflect technical manuals much more explicitly and intentionally than do CCSS (see here: (see http://core2class.blogspot.com/2013/09/the-buzz-over-technical.html). Finally, any good standards document should stipulate what students should know, but it’s also helpful to give teachers some suggestions so they understand how to operationalize the standards. The IN standards do this–helpfully, by the way–for every standard! Keep in mind that in the examples you give from CCSS math, don’t “dictate” pedagogy in the same way that the IN standards don’t. They are simply ways to help teachers operationalize the standards. From your piece: “Fluently add and subtract within 1000 using strategies and algorithms based on place value, properties of operations, and/or the relationship between addition and subtraction.” Teachers can choose how to apply standards like this in any way they choose.
Lots of ire over CCSS, but has anyone done some specific comparisons between the two standards frameworks? I think in doing so, there are lots of current CCSS criticisms that also pertain to IN Stds. Let’s be honest about this.
I don’t know about IN but the comparison has bee done for GA by more than one person and CCSS do not measure up. http://marykaybacallao.jimdo.com/common-core-math-standards/
http://www.senatorligon.com/Georgia%20-%20Math%20Standards%20Comparison%20Report.pdf
http://www.senatorligon.com/Georgia-%20Comparison%20of%20English%20Language%20Arts%20Standards.pdf
Sandra Stotsky (who was on the CCSS validation commitee)refused to sign off on the standards because they were not good. And the math expert on the validation committee refused to sign off on them as well. Not to mention the joint statement from FIVE HUNDRED early childhood professionals showing how the CCSS are NOT developmentally appropriate. http://www.edweek.org/media/joint_statement_on_core_standards.pdf
So yes, people how are opposed have done THEIR homework, have you? Lets be honest about this.
Thanks for the comment back.
Two counterpoints:
(1) Stotsky’s objections are thoughtful and should be taken into account. I think debating English teachers’ role in teaching informational text within the English curriculum is worth a discussion. However, let’s keep in mind that there are many others that served on the ELA validation committee who are in favor of the standards (e.g. Carol Jago who was Stotky’s partner in evaluating state ELA standards for Fordham). In addition, there are many curriculum experts out there who think CCSS ELA is a big step forward in terms of teaching and assessment (e.g. Wiggins, Shanahan, Marzano, McTighe, Lemov). Is it fair for us to engage in the specifics of this debate (by looking at the standards themselves) instead of simply citing Stotksy’s criticisms as evidence of why CCSS ELA is inferior to the IN ELA Standards? Stotky is ONE expert but not THE expert on all things related to English standards.
(2) I think it’s interesting to hear this growing argument coming from CCSS opponents that CCSS ELA is not developmentally appropriate. Isn’t the implication, then, that CCSS is rigorous…TOO rigorous in fact?!! You can’t have it both ways. You can’t accuse the standards of being too low-minded on the one hand then call them too rigorous on the other.
I enjoy the debate. Thanks!
Two points, Stotsky isn’t the only person who is against Common Core. The only English professor on the CC feedback committee was Mark Bauerlain and he is not on board with CC. Please read their joint paper How the Common Core ELA Standards Place College-Readiness at Risk here http://pioneerinstitute.org/download/how-common-cores-ela-standards-place-college-readiness-at-risk/
I use her research quite often because she has a unique perspective. I’m not sure if you are aware, but she helped Indiana rewrite their standards which placed them in the top three of the country. She is very familiar with the similarities and differences between IAS and CC. Sitting on the validation committee for CC is also a perspective many don’t have. This combination of experience is hard to match in finding adequate sources on CC, thus many use her work.
I’m not sure if you have watched the video of the child psychologist who evaluated the developmental appropriateness of the standards, but I would encourage you to do so. Rigor doesn’t mean giving children problems they mentally can’t process yet. Abstract thinking is not possible for many young children because their brain hasn’t developed the processes yet. It is like telling a child to grow a beard. Until their body is ready, it won’t happen. Forcing kindergarteners and first graders to “think abstractly and quantitatively” will result in frustration for both students and teachers.
Link to video: http://www.youtube.com/watch?v=vrQbJlmVJZo
Let’s just follow Mr. Daniel’s logic all the way through. If states cannot set their ‘educational bars’ for high school students, then shouldn’t someone be setting the common ‘educational bar’ for Purdue, MIT, Cal Tech and Stanford relative to the level of achievement for Electrical Engineers. I don’t see that happening. Somehow it is missed upon me that University teachers and administrators can successfully set their own bars, but K-12 teachers and administrators with their decades of experience and master’s degrees and doctorates are somehow unable to determine appropriate bars for their students.
Maybe that is the do as I say, not as I do logic.
Only AFTER the Education Colleges start setting the academic bar higher for those who want to be teachers. Haven’t you heard the phrase “Those who can, do; those who can’t, teach.”
I take offense to your comment. I taught public school for 7 years. I went to college for 10 years. I have my elementary degree, special education degree, English as a Second Language Degree, as well as a Masters of Education…..I could have been a veterinarian if I wanted to be, but I DID NOT want to be. I LOVE teaching kids and that is why I chose to be a teacher. I can do whatever I set my mind to, I chose to be a teacher because I love it. Not because I could not do whatever….so I became a teacher. RUDE!!!!
The idea of teaching with multiple strategies is so children can use whatever works best for them. Those are examples. I am a homeschooling mother who is also a senior in an early childhood education program. The reason many children are behind in mathematics is because they are lacking number sense. Rote memorization of facts may work for some children, but not for all. Rote memorization doesn’t allow children to gain that number sense. By using different types of manipulatives and strategies, children can develop their own numbers sense and see relationships that they previously did not. This will allow them to think critically about more complex problems. It is proven that children learn best by constructing their own knowledge, not by being told what to know.
I think you may misunderstand me. I don’t object to teaching the underlying concepts, but how long should students be allowed to develop their own strategies? After awhile they need to learn the most efficient methods. Under CC, they allow two years to use these strategies for addition/subtraction before they teach the standard algorithm. Not just those who don’t understand- everyone. Math is a progression to algebra. Slowing them down by focusing on student developed strategies won’t get them there by 8th grade like all high-performing countries do.
Kellie, the way CC is being implemented does not allow for students to pick which strategy they like best or is best for them. It requires them to analyze and implement multiple strategies, whether they find it intuitive/helpful or not. The and/or in the standards is being considered an and by curricula (ie. test authors). Erin, I do not see efforts to encourage or allow students to “develop their own strategies.” I actually think that would be better than what we have now-students could reflect on and explore numbers in a meaningful way. If the common core is going to promote constructivist results like critical thinking and solving meaningful problems, it needs to allow teachers autonomy to let students (especially lower grades) build number sense in an organic way (with input from early childhood development experts and teachers) on a less specific timeline, using the strategy they feel comfortable with per their own learning styles and abilities.
Teaching multiple algorithms has its advantages. It’s a great way to force parents to pay for private tutors.
Indeed, they say the number of Kumon and Sylvan Learning Centers increases dramatically when school implement fuzzy math.
I have been an Algebra teacher for nearly 25 years and I have studied the Common Core Standards. Algorithms are taught. They just aren’t where students should begin. They should begin with an understanding of what it means to add ( or multiply or divide) 2-digit(or higher) numbers. If students really understand those things in elementary school, then they make Algebra so much more accessible. Understanding before algorithms is key.
I agree that conceptual understanding is important, but how long do you wait to introduce the standard algorithm? Under CC, kids will spend years working on addition and subtraction before the standard algorithm is required in grade 4. In high-performing countries and states like Massachusetts (number one on NAEP and TIMMS) they start using the standard algorithm in second grade. How long do you think it should be delayed?
Good write up 10/10 :).